![]() 18 Dec 2023
18 Dec 2023
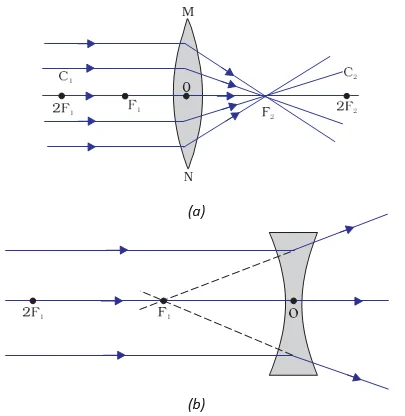
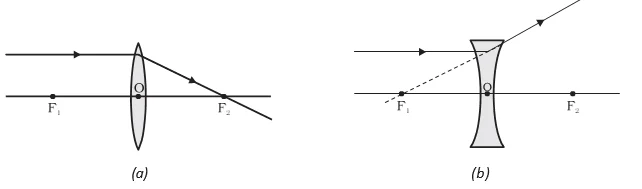
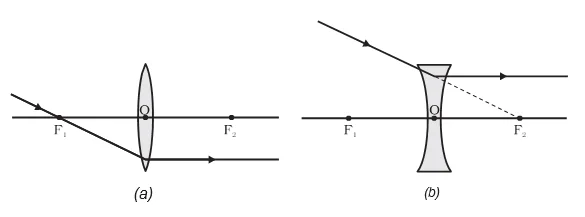
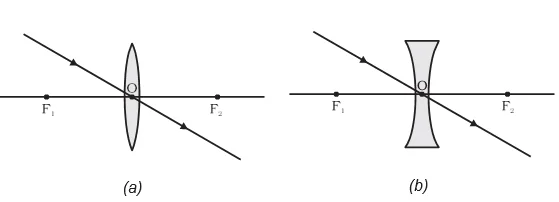
Spherical Lens Image Formation and Optical Variations:

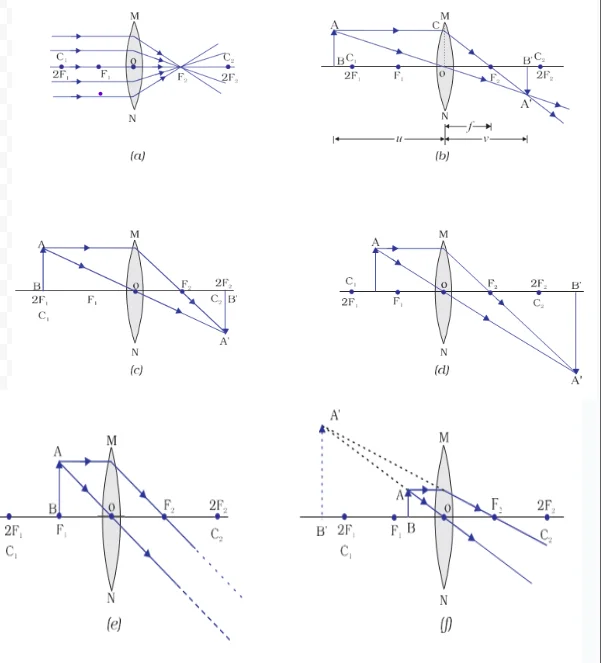
In conclusion it can be said that a concave lens will always give a virtual, erect and diminished image, irrespective of the position of the object.
Spherical Lens Sign Convention: Optical Measurements and Focal Insights
Spherical Lens Formula: Understanding Relationships in Optics:
Spherical Lens Magnification: Optical Enlargement and Relationships
![]()
Spherical Lens Power Dynamics: Optical Convergence and Divergence
![]()
|
Designing a lens
|
|---|
<div class="new-fform">
</div>