PWOnlyIAS
November 12, 2024 06:44
2949
0
The UPSC IFS Mains Statistics Optional Paper Syllabus, trends, and success rates are provided here. Making an informed decision on choosing Statistics as your optional subject with insights on challenges and advantages
UPSC IFS Mains Statistics Optional Paper: The UPSC Indian Forest Service (IFS) Mains Statistics Optional Paper is one of the specialized subjects that candidates with a background in statistics, mathematics, or related fields often choose. This optional consists of two papers, each carrying 200 marks, making a total of 400 marks for the Statistics optional. Statistics optional is known for its structured and analytical nature, with topics covering both theoretical and applied aspects of the field. Key areas include probability, statistical inference, sampling theory, applied statistics, and data analysis techniques. With a well-defined syllabus and scoring potential, the Statistics optional offers candidates an opportunity to showcase their analytical skills and quantitative reasoning in the IFS Mains Examination.
In the UPSC IFS Mains exam, the Statistics optional subject consists of two papers, specifically Paper I and Paper II. Each of these papers carries a weightage of 200 marks, summing up to a total of 400 marks for this optional subject. Among the various optional subjects offered by UPSC in IFS, Statistics Optional is one of the 14 optional subjects available to candidates.
| Statistics | Syllabus of Statistics Optional |
| Paper I | Probability- Sample space and events, probability measure and probability space, random variable as a measurable function, distribution function of a random variable, discrete and continuous-type random variable probability mass function, probability density function, vector-valued random variable, marginal and conditional distributions, stochastic independence of events and of random variables, expectation and moments of a random variable, conditional expectation, convergence of a sequence of random variable in distribution, in probability, in p-th mean and almost everywhere, their criteria and inter-relations, Borel-Cantelli lemma, Chebyshev’s and Khinchine’s weak laws of large numbers, strong law of large numbers and Kolmogorov’s theorems, Glivenko-Cantelli theorem, probability generating function, characteristic function, inversion theorem, Laplace transform, related uniqueness and continuity theorems, determination of distribution by its moments. Linderberg and Levy forms of central limit theorem, standard discrete and continuous probability distributions, their interrelations and limiting cases, simple properties of finite Markov chains.
Statistical Inference- Consistency, unbiasedness, efficiency, sufficiency, minimal sufficiency, completeness, ancillary statistic, factorization theorem, exponential family of distribution and its properties, uniformly minimum variance unbiased (UMVU) estimation, RaoBlackwell and Lehmann-Scheffe theorems, Cramer-Rao inequality for single and several-parameter family of distributions, minimum variance bound estimator and its properties, modifications and extensions of Cramer-Rao inequality, Chapman-Robbins inequality, Bhattacharyya’s bounds, estimation by methods of moments, maximum likelihood, least squares, minimum chi-square and modified minimum chi-square, properties of maximum likelihood and other estimators, idea of asymptotic efficiency, idea of prior and posterior distributions, Bayes’ estimators. Non-randomised and randomised tests, critical function, MP tests, Neyman-Pearson lemma, UMP tests, monotone likelihood ratio, generalised Neyman-Pearson lemma, similar and unbiased tests, UMPU tests for single and several-parameter families of distributions, likelihood rotates and its large sample properties, chi-square goodness of fit test and its asymptotic distribution. Confidence bounds and its relation with tests, uniformly most accurate (UMA) and UMA unbiased confidence bounds. Kolmogorov’s test for goodness of fit and its consistency, sign test and its optimality. Wilcoxon signed-ranks test and its consistency, Kolmogorov-Smirnov two-sample test, run test, Wilcoxon-Mann-Whiltney test and median test, their consistency and asymptotic normality. Wald’s SPRT and its properties, OC and ASN functions, Wald’s fundamental identity, sequential estimation. Linear Inference and Multivariate Analysis- Linear statistical models, theory of least squares and analysis of variance, GaussMarkoff theory, normal equations, least squares estimates and their precision, test of significance and interval estimates based on least squares theory in one-way, two-way and three-way classified data, regression analysis, linear regression, curvilinear regression and orthogonal polynomials, multiple regression, multiple and partial correlations, regression diagnostics and sensitivity analysis, calibration problems, estimation of variance and covariance components, MINQUE theory, multivariate normal distribution, Mahalanobis D2 and Hotelling’s T2 statistics and their applications and properties, discriminant analysis, canonical correlations, one-way MANOVA, principal component analysis, elements of factor analysis. Sampling Theory and Design of Experiments- An outline of fixed-population and super-population approaches, distinctive features of finite population sampling, probability sampling designs, simple random sampling with and without replacement, stratified random sampling, systematic sampling and its efficacy for structural populations, cluster sampling, two-stage and multi-stage sampling, ratio and regression, methods of estimation involving one or more auxiliary variables, two-phase sampling, probability proportional to size sampling with and without replacement, the Hansen-Hurwitz and the Horvitz-Thompson estimators, non-negative variance estimation with reference to the Horvitz-Thompson estimator, non-sampling errors, Warner’s randomized response technique for sensitive characteristics. Fixed effects model (two-way classification) random and mixed effects models (two-way classification per cell), CRD, RBD, LSD and their analyses, incomplete block designs, concepts of orthogonality and balance, BIBD, missing plot technique, factorial designs : 2n, 32 and 33, confounding in factorial experiments, split-plot and simple lattice designs. |
| Paper II | Industrial Statistics– Process and product control, general theory of control charts, different types of control charts for variables and attributes, X, R, s, p, np and c charts, cumulative sum chart, V-mask, single, double, multiple and sequential sampling plans for attributes, OC, 47 ASN, AOQ and ATI curves, concepts of producer’s and consumer’s risks, AQL, LTPD and AOQL, sampling plans for variables, use of Dodge-Romig and Military Standard tables. Concepts of reliability, maintainability and availability, reliability of series and parallel systems and other simple configurations, renewal density and renewal function, survival models (exponential), Weibull, lognormal, Rayleigh, and bath-tub), different types of redundancy and use of redundancy in reliability improvement, problems in life-testing, censored and truncated experiments for exponential models. II.
Optimization Techniques- Different, types of models in Operational Research, their construction and general methods of solution, simulation and Monte-Carlo methods, the structure and formulation of linear programming (LP) problem, simple LP model and its graphical solution, the simplex procedure, the two-phase method and the M-technique with artificial variables, the duality theory of LP and its economic interpretation, sensitivity analysis, transportation and assignment problems, rectangular games, two-person zero-sum games, methods of solution (graphical and algerbraic). Replacement of failing or deteriorating items, group and individual replacement policies, concept of scientific inventory management and analytical structure of inventory problems, simple models with deterministic and stochastic demand with and without lead time, storage models with particular reference to dam type. Homogeneous discrete-time Markov chains, transition probability matrix, classification of states and ergodic theorems, homogeneous continuous-time Markov chains, Poisson process, elements of queueing theory, M/M/1, M/M/K, G/M/1 and M/G/1 queues. Solution of statistical problems on computers using well known statistical software packages like SPSS. Quantitative Economics and Official Statistics- Determination of trend, seasonal and cyclical components, Box-Jenkins method, tests for stationery of series, ARIMA models and determination of orders of autoregressive and moving average components, forecasting. Commonly used index numbers-Laspeyre’s, Paashe’s and Fisher’s ideal index numbers, chain-base index number uses and limitations of index numbers, index number of wholesale prices, consumer price index number, index numbers of agricultural and industrial production, tests, for index numbers like proportionality test, time-reversal test, factor-reversal test, circular test and dimensional invariance test. General linear model, ordinary least squares and generalised least squires methods of estimation, problem of multicollinearity, consequences and solutions of multicollinearity, autocorrelation and its consequences, heteroscedasticity of disturbances and its testing, test for independence of disturbances, Zellner’s seemingly unrelated regression equation model and its estimation, concept of structure and model for simultaneous equations, problem of identification-rank and order conditions of identifiability, two-stage least squares method of estimation. Present official statistical system in India relating to population, agriculture, industrial production, trade and prices, methods of collection of official statistics, their reliability and limitation and the principal publications containing such statistics, various official agencies responsible for data collection and their main functions. IV. Demography and Psychometry- Demographic data from census, registration, NSS and other surveys, and their limitation and uses, definition, construction and uses of vital rates and ratios, measures of fertility, reproduction rates, morbidity rate, standardized death rate, complete and abridged life tables, construction of life tables from vital statistics and census returns, uses of life tables, logistic and other population growth curves, fitting a logistic curve, population projection, stable population theory, uses of stable population and quasi-stable population techniques in estimation of demographic parameters, morbidity and its measurement, standard classification by cause of death, health surveys and use of hospital statistics. Methods of standardisation of scales and tests, Z-scores, standard scores, scores, percentile scores, intelligence quotient and its measurement and uses, validity of test scores and its determination, use of factor analysis and path analysis in psychometry. |
UPSC Statistics Optional Question Papers from 2018 to 2022 are readily available for aspirants seeking to enhance their preparation. We provided access to the UPSC IFS Statistics optional question papers in PDF format, an invaluable resource for your preparation.
| Year | Paper of Statistics Optional |
| UPSC IFS Statistics Optional Question Paper 2018 | Download Paper I |
| UPSC IFS Statistics Optional Question Paper 2019 | Download Paper I |
| UPSC IFS Statistics Optional Question Paper 2020 | Download Paper I |
| UPSC IFS Statistics Optional Question Paper 2021 | Download Paper I |
| UPSC IFS Statistics Optional Question Paper 2022 | Download Paper I |
| UPSC IFS Statistics Optional Question Paper 2023 | Download Paper I |
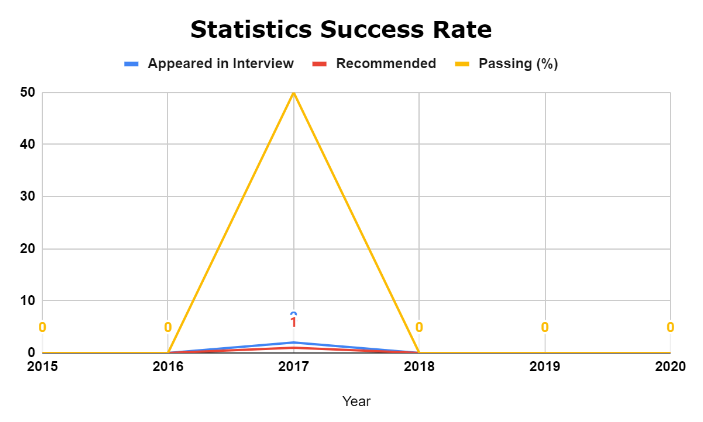
The table below offers a comprehensive overview of the success rate achieved by candidates who opted for Statistics Paper as their optional.
| Year | Number of Candidates | ||
| Appeared in Interview | Recommended | Passing (%) | |
| 2015 | 0 | 0 | 0 |
| 2016 | 0 | 0 | 0 |
| 2017 | 2 | 1 | 50 |
| 2018 | 0 | 0 | 0 |
| 2019 | 0 | 0 | 0 |
| 2020 | 0 | 0 | 0 |
By examining the past papers of the Statistics Optional, we can identify a trend in the types of questions asked. There is also a noticeable shift in the themes of the questions. Understanding the UPSC IFS Statistics Optional Question Paper Trend Analysis is advantageous, as it allows candidates to streamline their preparation effectively and enhance their performance in this optional subject.
Please note that before selecting Statistics as your optional subject, carefully weigh these disadvantages against the advantages and consider your own background, interests, and the time you can dedicate to preparation.
| Must Read | |
| NCERT Notes For UPSC | UPSC Daily Current Affairs |
| UPSC Blogs | UPSC Daily Editorials |
| Daily Current Affairs Quiz | Daily Main Answer Writing |
| UPSC Mains Previous Year Papers | UPSC Test Series 2024 |
.png)
Connect with our experts to get free counselling & start preparing
Learn From India's Best Faculty
Learn From India's Best Faculty
<div class="new-fform">
</div>
Latest Comments